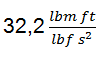1. If a US gallon has a volume of 0.134 ft3 and a human mouth has a volume of 0.900 in3, then how many mouthfuls of water are required to fill a 5.00 US gallon can?
2. Identify
whether you would perform the following unit conversions by definition, by conversion factors, by geometry, or by scientific law.
a. How many square miles in a square kilometer?
1.000
mile = 1.609 km
1
mile square =12x 1.609342 [mile2] [km2/mile2]
=2.589 km2
b. How many microfarads in a farad?
1F =
1 x 106 [F][µF/F] = 106 µF
c. What is the weight on Earth in N of an object
with a mass of 10.0 kg
W=m
x g
W=10.0
kg x 9.81 m/s2 = 98 kg m/s2 = 98.0 N
d. How many square miles on the surface of the
Earth?
Known:
Radius of earth = 6371 km
3. The height of horses from the ground to their shoulder is still measured in the old unit of hands. There are 16 hands in a fathom and 6.0 feet in a fathom. How many feet high is a horse that is 13 hands tall?
4.An acre originally was defined as the amount of land that an oxen team could plow in a day. Suppose a team could plow 0.4 hectare per day, where a hectare is 104 m2. There are 1609 meters in a mile. How many acres are there in a square mile?
5. There are 39 inches in a meter. What is the area
in the SI system of the skin of a spherical orange that is 4.0 inches in
diameter?
6. There are 39 inches in a meter. What is the volume in the Engineering
English system of a spherical apple that is 10. cm (note the decimal point
here) in diameter?
7. If the pressure in the tire on your car is 32.0
lbf/in2 (or psi), what is its pressure in SI units?
8. Suppose
the mass in Example 2.1 was 50.0 slugs. What would be its weight in lbf (pounds
force)?
9. What would the 5.00 slug mass in Example 2.1 weigh on the Moon where the acceleration of gravity is only 1/6 of that on Earth?
Mass doesn't depend on the gravity, so the mass on the moon is still the same, 5.00 slug mass.
10. What would be the weight of the body in Example 2.3 on the Moon
where the acceleration of gravity is just gmoon = 1.64 m/s2?
Example 2.4 What is the weight in newtons of a mass of 0.102 kg?
11. What would be the weight of the body in Example 2.3 on the Moon where the acceleration of gravity is just g Moon ¼ 1.64 m/s2?
12. What force would be necessary in Example
2.4 if the mass were 735 lbm?
Example
2.4 What is the force necessary to
accelerate a mass of 65.0 lbm at a rate of 15.0 ft/s2? Since the problem is
stated in English units, assume the answer is required in these units. Equation
(2.7) is the principle used here:
13. What is the value and units of gc
in the Engineering English system on the Moon?
14. Acceleration is sometimes measured in g’s, where
1.0 g = 9.8 m/s2. How many g’s correspond to the steady acceleration of a car
doing “zero to sixty”15 in 10.0 seconds?
15. What is your mass in kilograms divided by your weight in pounds? Do you have to step onto a scale to answer this question? How did you answer the question?
16. If power (measured in W, or watts) is defined as work (measured in J, or joules) performed per unit time (measured in s), and work is defined as force (measured in N or newtons) distance (measured in m) and speed is defined as distance per unit time (measured in m/s), what is the power being exerted by a force of 1000. N on a car traveling at 30. m/s? (Assume force and speed are in the same direction, and treat all numbers as positive.)
17. A rocket sled exerts 3.00 x 104
N of thrust and has a mass of 2.00 x 103
kg. In how much time does it do “zero to sixty”? How many g’s (see Exercise 14)
does it achieve?
18. A person pushes a crate on a frictionless
surface with a force of 100. lbf. The crate accelerates at a rate of 3.0 feet
per second2. What is the mass of the crate in lbm?
19. The force of gravity on the Moon is one-sixth
(i.e., 1/6.0) as strong as the force of gravity on Earth. An apple weighs 1.0 N
on Earth.
(a) What is the mass of the apple on the Moon, in lbm?
(b) What is the
weight of the apple on the Moon, in lbf? (Conversion factor: 1.00 kg = 2.20
lbm)
20. How
many lbf does it take for a 4.0 x 103 lbm car to achieve 0 to 60 mph
in 10. seconds?
21. Suppose a planet exerted a gravitational force at its surface that was 0.6 the gravitational force exerted by Earth. What is gc on that planet?
The force is the same because it is just influenced by the mass and the acceleration of the object, that is
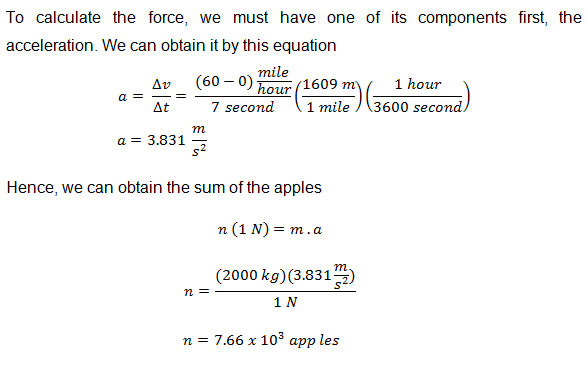
22. Suppose you were going to accelerate a 2000. kg car by the Rube Goldberg contraption shown in the following figure. The fan (A) blows apples (C) off the tree (B) into the funnel and thus into the bag (D). The bag is pulled downward by the force of gravity (equal to the weight of the apples in the bag), and that force is transmitted via the pulley (E) to accelerate the car (F). About how many apples each weighing 1.00 N would have to fall into the bag in order to achieve 0 to 60.0 mph in 7.00 seconds? Assume the filled bag applies a constant force to the car, equal to the weight of the apples in the bag.